Using a modification of the optimal f money management approach, this article will show you how to determine a new fractional value of capital to invest in each transaction in order to optimize returns while adhering to a drawdown restriction.
We have developed and deployed evaluation software to trading systems that employ money management strategies during the past four years, and we have investigated the effects of these techniques on trading systems. One of the results of that work is a new money management method called secure f, and in this article we’ll show you how to discover a fresh fractional amount of cash to invest in each transaction in order to optimize returns while keeping drawdown to a minimum. Depending on the trader’s chosen maximum allowed drawdown, Secure F can be either a conservative or an aggressive strategy. It is a version of Ralph Vince’s portfolio management formulas, which established the optimal f approach. Secure f varies from optimum f in that it uses data on the pricing of the underlying security and considers historical drawdowns.
By determining the best percentage of capital to invest in each trade, the optimal f money management approach can be employed to enhance and maximize system performance. Based on a series of previous trades, this technique calculates the percentage of equity that would have been put in a trade to produce the best return. It can be helpful to know what would have been the ideal amount to invest in each situation because traders can utilize a range of money management techniques. The Kelly formula, which determines the percentage of your capital to trade with when the quantities earned and lost are not equal, is where the idea of a percent or fractional strategy originates from:
f = ((b+1)p – 1))/bb = Ratio of the size won on a winning bet to the size lost on a losing bet
p = Probability of a winning bet
A straightforward illustration would be if you made or lost the same amount on three bets, two of which won and one of which lost (1, 1, 0):
f = ((1+1)0.666-1)1 = 0.33333
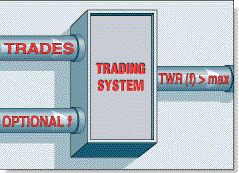
This formula finds the value of f. When there are just two possible outcomes, this formula is appropriate. There are several possible outcomes for traders. When Vince introduces optimal f, we must maximize what Vince refers to as terminal wealth relative (TWR) in order to determine optimal f’s value. The issue can be stated as follows:
TWR(f) -> max
where TWR(f)=(HPR1(f))((HPR2(f))(…(HPRn(f))
HPRi(f)=1+(f((-Return on the trade i)/(Return on the worst losing trade))
HPR = Holding period return
The optimization issue that was resolved by optimum f is shown in the following figure. The final equity is maximized by optimal f by investing the proper amount in each trade, as shown by the description of optimal f and Figure 1, respectively. This sum represents f% of the equity that is in place at the moment the trade is started. The computations are then applied to a collection of past transactions to determine the value of optimal f. Trade history must be lucrative in order for any strategy, including optimum f, to change a losing strategy into a winning one. The more final equity is generated by the implementation of optimal f, the longer it is used.
Depending on the level of risk they are ready to take, traders can use a secure f money management approach to maximize their return.